This course provides an introduction to the methods of nonlinear dynamics and mathematical physics used to analyze and explain patterns in Physics, Chemistry, and Biology. Central tools of applied mathematics are mastered on a number of analytically solvable examples, ranging from well established instabilities to recently discovered pattern-forming phenomena. Depending on the number of participants and interest, the course can be supplemented by mini-projects.
Core contents
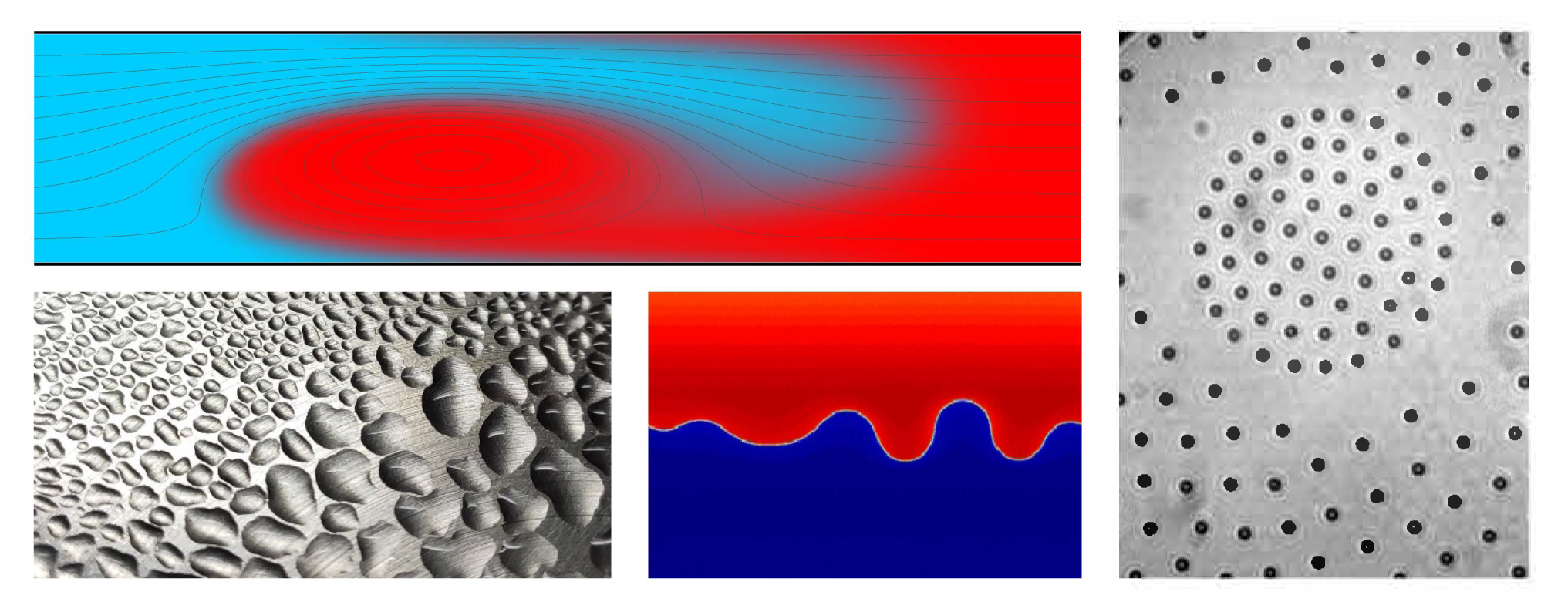
- A quick and easy intro into fluid mechanics
- Classical hydrodynamic instabilities
- Biochemical instabilities and front propagation
- Absolute and convective instabilities, global mode
- Dynamic pattern-forming behavior in colloidal soft matter systems
- Extra topics: Patterns in colloidal liquid crystals, dynamic mode locking
Enrolment details
- Kursverantwortliche/r: Arthur Straube
Semester: SoSe 2021